PhD Thesis: Global Parametrization Algorithms for Quadmeshing

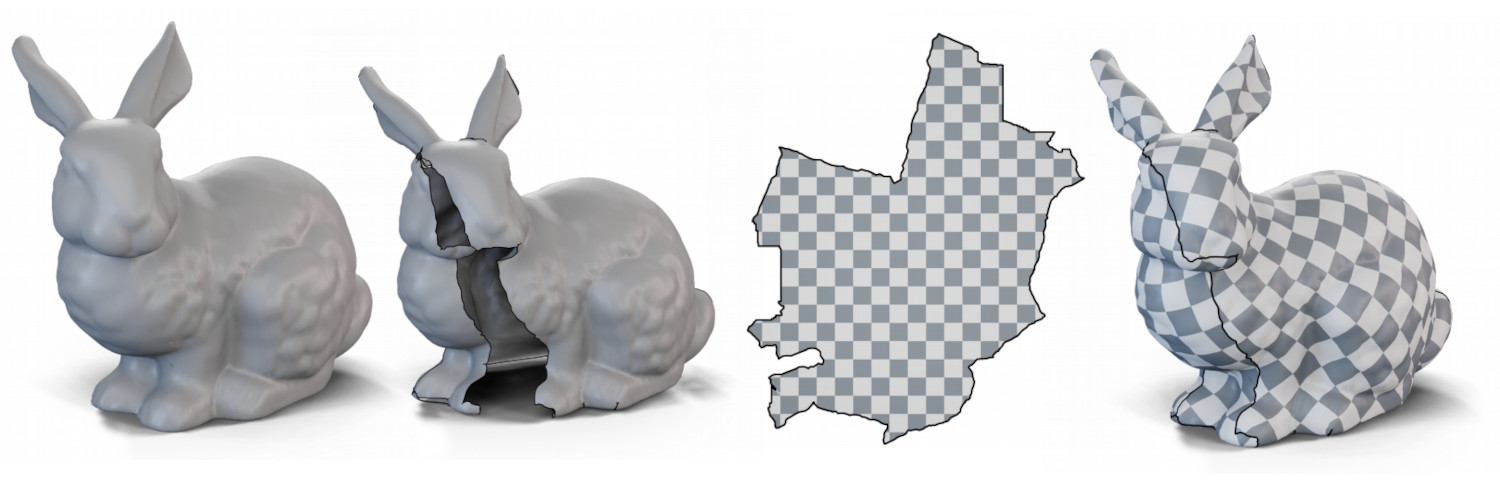
Quadrangular meshes are a central data structure in the domain of geometry processing, with applications ranging from computer graphics to numerical simulation. A promising approach for automatic generation of high quality quadmeshes relies on the observation that they consist in a deformation of the regular grid almost everywhere, except at a few singular vertices. Thanks to the computation of a parametrization, that is to say a flat representation, of the surface to be meshed, it is then possible to overlay a grid to be lifted back as a quad mesh. For quads to be extracted from this surface parametrization, it has to be seamless, that is to say satisfy a set of alignment constraints on its boundary and its cuts. This needs a quantization as integer values of some of its degrees of freedom. These constraints are usually enforced progressively in a well-studied pipeline of operations, consisting in the computation of a smooth frame field, defining the future singular vertices of the mesh, an integration phase from which a rotationally seamless parametrization is recovered, and a quantization step to determine the translational degrees of freedom.